A 9-Year-Old Came Up With This Weirdly Tricky Math Problem. Can You Solve It?

A system of nonlinear equations is harder to solve than you think—and more of a puzzle than a math problem.
Puzzles like this require you to look and find the one loose end you can pull to unravel the rest.
This kid is awesome.
Novelist Celeste Ng has shared a head-scratching system of equations that her 9-year-old son apparently made up over dinner:
She’s right—using simultaneous equations does get really complicated, and if you’d like to send me your work for the solution, I’d love to see it. But the system is also kind of a trick. Like a sudoku or crossword puzzle, there’s one easier clue that lets you begin to unravel the rest.
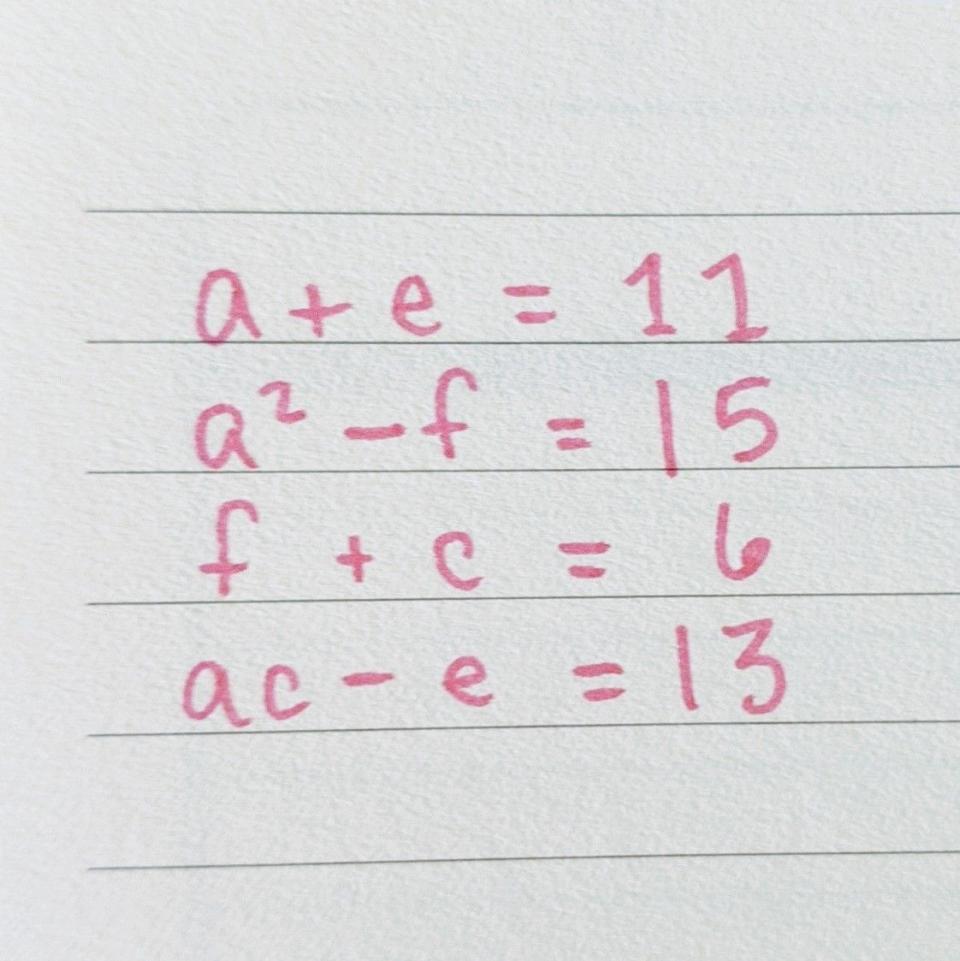
Can you see it? The secret this time is in the second line.

Having a squared term is like a golden ticket. We immediately know that we’re dealing with one of just a handful of squares that could fit the bill.

There could be negative and non-integer solutions to all these things—hey, let me know if you solve them out!—but that didn’t seem like something even a sneaky and gifted 9-year-old kid would pull. And if our a value were anything bigger than about 5, the f value would be way out of skew with the others.
I immediately thought “a is 4 and f is 1,” because this isn't my first math rodeo and I suspect it’s not yours either. The geometry teacher gives you class examples of Pythagorean triples like 3, 4, 5 and 5, 12, 13—not the ugly decimal values you find in real life. When we start to substitute those two values back into the equation system, everything else falls into place.

So f = 1, making c = 5. If a = 4, then e = 7. Our solution is {4, 5, 7, 1}.
Why is this system so hard to solve by traditional algebra? It’s because these aren’t simple linear equations. As soon as our hero multiplied a × a and then a × c, things got complicated. A squared term indicates a quadratic equation—in this example it’s even the special case of the difference of two squares, where the middle term falls away during cross multiplication. And the ac – e one is more like bilinear, where two variables are multiplied together, which isn’t quadratic complicated, but is trickier to solve than a straightforward linear equation set.
I’m a big fan of Ng and her burgeoning math demi-villain, whose recent specific interests include Monopoly and, of course, Lego bricks. Last fall, he fixed his mom’s tape dispenser.
Honestly, challenging math puzzles are a big step toward “lawful good” for this smart kid. Kudos to his novelist mom, too, for working on math problems at dinner and encouraging his creativity, which will hopefully help to continue to take down the pretend barrier between being creative and being mathematical.
You Might Also Like